We will finish this chapter with a last example: finding the real roots of a quadratic equation ax2+bx+c = 0. We will start by considering the case where a equals 0:

The preceding transcript contains the definition of a function L(b,c) that solves this particular case.The general case can then be treated as follows:
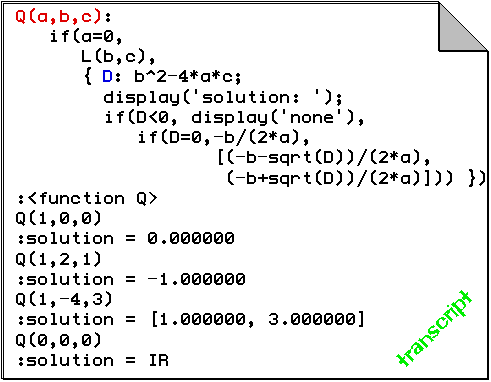
The function
Q(a,b,c)
defers to L
whenever a
is zero; in the other case a discriminant
D
is computed and depending on its value the existence of a double root
-b/2a or two distinct roots (-b ± ÷D)/2a
is reported.